Inversion in Euclidean Circles

  In order to define congruence in the Poincaré model and verify the axioms of congruence, we must study the operation of inversion in a Euclidean circle. This operation is the process of reflecting across a line in the Poincaré disk model of the hyperbolic plane.

Let be a circle of radius r and center O. For any point the inverse  P' of P with respect to the circle is the unique point so that . In this case is called the circle of inversion. 

These are Euclidean geometry theorems about circles, so we may use the facts that we know about Euclidean geometry.

Proposition 15.1: For and as above,

1. P=P' if and only if .
2. If then , and vice versa.
3. (P')'=P.

Proposition 15.2: Let and let TU be the chord through P perpendicular to . Then P'=P(TU), the pole of TU, i.e., the intersection of the tangents to at T and U.  

Proof: Suppose the tangent to at T cuts at the point P'. The right triangle is similar to right triangle OTP' (since they have in common and the angle sum is ). Hence corresponding sides are proportional. Since , we get that

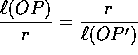
which shows that P' is the inverse to P. Reflect across the line , and we see that the tangent to at U also passes through P', so that P' is the pole of TU.

Proposition 15.3: If P is outside of , let Q be the midpoint of OP. Let be the circle of radius QP centered at Q. Then

1. .
2. and are tangent to .
3. .

Proof: By the circular continuity principle, and do intersect in two points T and U. Since and are inscribed in semicircles of , they are right angles. Therefore and are tangent to . If TU intersects OP in a point P', then P is the inverse of P', by the previous proposition. Thus, P' is the inverse of P in .

The next proposition shows how to construct the Poincaré line joining two ideal points--the line of enclosure.  Its proof shows that in the previous figure is indeed a right angle, as we needed.

Proposition 15.4: Let T and U be points on that are not contained on a the same diameter, and let P be the pole of TU. Then

1. ,
2. ,
3. , and
4. the circle with center P and radius PT cuts orthogonally at T and U.

n

Proof: By definition of pole, and are right angles, so by the Hypotenuse-Leg criterion, . Therefore, and . The base angles and of the isosceles triangle are thus congruent, and the angle bisector is perpendicular to the base TU. The circle is then well-defined because and intersects orthogonally by the hypothesis that and are tangent to .

Let P be a point in the plane and a circle with center O. The power of P with respect to is defined to be

 

Proposition 15.5: Assume and assume that two lines through P intersect in points and . Then

1. .
2. If one of these lines through P is tangent to at T, then .

Proposition 15.6: Let and , the center of . Let be a circle through P. is orthogonal to if and only if passes through P', the inverse of P with respect to .  

Proposition 15.7: Let have radius r, have radius t and let P be the center of . is orthogonal to if and only if , where the power is computed with respect to .  

Let O be a point and k>0. The dilation with center O and ratio k is a mapping of the Euclidean plane that fixes O and maps every point to a unique such that . Call this map . 

Proposition 15.8: Let be a circle with center and radius s. maps to a circle with center and radius . If , the tangent to at is parallel to the tangent to at Q.

Proof: Choose rectangular coordinates so that O is the origin. Then the dilation is given by the mapping . The image of the line have equation ax+by=c is the line having equation , so the image is parallel to the original line. In particular, is parallel to , and their perpendiculars at Q and , respectively, are also parallel. If has equation , then has equation .

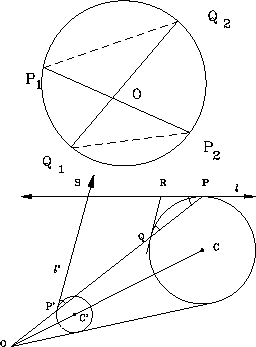
Proposition 15.9: Let be the circle of radius r centered at O and let be the circle of radius s centered at C. Assume O lies outside ; let p=Pw(O) with respect to and let . The image of under inversion in is the circle of radius whose center is . If and P' is the inverse of P with respect to , then the tangent t' to at P' is the reflection across the perpendicular bisector of PP' of the tangent to at P.

Corollary is orthogonal to if and only if is mapped to itself by inversion in .

Proposition 15.10: Let be a line so that . The image of under inversion by is a punctured circle with missing point O. The diameter through O of the completed circle is perpendicular to .

Proposition 15.11: Let be a circle passing through the center O of . The image of minus O under inversion in is a line so that and is parallel to the tangent to at O.

Proposition 15.12: A directed angle of intersection of two circles is preserved in magnitude by an inversion. The same applies to the angle of intersection of a circle and a line or the intersection of two lines.

Proposition 15.13: Let be orthogonal to . Inversion in maps onto and the interior of onto itself. Inversion in preserves incidence, betweenness and congruence in the sense of the Poincaré disk model.