Skip to main content

Section 14.3 The Number \(e\)

What is \(\pi\text{?}\) It's a number that starts with 3.14, but it goes on forever and never repeats. So, mathematicians usually just write \(\pi\text{,}\) rather than rounding some number of decimal places. The number \(e\) is the same idea.

Essentially, the whole point of this section is that \(e\) is a number, so we treat it like any other number. You don't need to know what the decimal is, but it will come in handy to know that it's a little bit less than 3.

Example 14.20.

What is the initial value and growth/decay rate of each of the following exponential functions?

  1. For \(f(x)=7e^x\text{:}\)

    • The initial value is just \(7\text{,}\) like we saw in Section 14.1.

    • The rate comes from the thing that's raised to the \(x\text{,}\) which in this case is \(e\text{.}\) That means that \(e=1+\text{rate(decimal)}\text{.}\) Solving for the rate, we get that the rate (as a decimal) is \(e-1\text{.}\) To convert to a percentage, we just need to mulitply by 100. So, the rate is \((e-1)100\)%. Since \(e\) is approximately 3, \(e-1\gt0\text{,}\) so this is a growth rate.

  2. For \(f(x)=0.2e^{-x}\text{:}\)

    • The initial value is just \(0.2\text{,}\) like we saw in Section 14.1.

    • The rate comes from the thing that's raised to the \(x\text{.}\) In this case, we need to separate the \(x\) from the negative that's in the exponent. Since \(e^{-x}=(e^{-1})^x\text{,}\) we have that the thing that's raised to the \(x\) is \(e^{-1}\text{,}\) which we can write as \(\frac{1}{e}\text{.}\) That means that \(\frac{1}{e}=1+\text{rate(decimal)}\text{.}\) Solving for the rate, we get that the rate (as a decimal) is \(\frac{1}{e}-1\text{.}\) To convert to a percentage, we just need to mulitply by 100. So, the rate is \(\left(\frac{1}{e}-1\right)100\)%. Since \(e\) is approximately 3,\(\frac{1}{e}\) is approximately \(\frac{1}{3}\text{,}\) so \(\frac{1}{e}-1\lt0\text{,}\) so this is a decay rate.

Checkpoint 14.21.

Find the initial value and growth/decay rate of each of the following exponential functions

  1. \(\displaystyle f(x)=9e^x\)

  2. \(\displaystyle g(x)=-7e^{13x}\)

  3. \(\displaystyle h(x)=99e^{-2x}\)

Answer.

  1. The initital value is \(9\text{,}\) and the growth rate is \((e-1)100\)%.

  2. The initial value is \(-7\text{,}\) and the growth rate is \((e^{13}-1)100\)%.

  3. The initial value is \(99\text{,}\) and the decay rate is \(\left(\frac{1}{e^2}-1\right)100\)%.

Solution.

  1. For \(f(x)=9e^x\)

    • The initial value is just \(9\text{,}\) like we saw in Section 14.1.

    • The rate comes from the thing that's raised to the \(x\text{,}\) which in this case is \(e\text{.}\) That means that \(e=1+\text{rate(decimal)}\text{.}\) Solving for the rate, we get that the rate (as a decimal) is \(e-1\text{.}\) To convert to a percentage, we just need to mulitply by 100. So, the rate is \((e-1)100\)%. Since \(e\) is approximately 3, \(e-1\gt0\text{,}\) so this is a growth rate.

  2. For \(g(x)=-7e^{13x}\)

    • The initial value is just \(-7\text{,}\) like we saw in Section 14.1.

    • The rate comes from the thing that's raised to the \(x\text{.}\) In this case, we need to separate the \(x\) from the 13 that's in the exponent. Since \(e^{13x}=(e^{13})^x\text{,}\) we have that the thing that's raised to the \(x\) is \(e^{13}\text{.}\) That means that \(e^{13}=1+\text{rate(decimal)}\text{.}\) Solving for the rate, we get that the rate (as a decimal) is \(e^{13}-1\text{.}\) To convert to a percentage, we just need to mulitply by 100. So, the rate is \((e^{13}-1)100\)%. Since \(e\) is approximately 3, \(e^{13}-1\gt0\text{,}\) so this is a growth rate.

  3. For \(h(x)=99e^{-2x}\)

    • The initial value is just \(99\text{,}\) like we saw in Section 14.1.

    • The rate comes from the thing that's raised to the \(x\text{.}\) In this case, we need to separate the \(x\) from the \(-2\) that's in the exponent. Since \(e^{-2x}=(e^{-2})^x\text{,}\) we have that the thing that's raised to the \(x\) is \(e^{-2}\text{,}\) which we can write as \(\frac{1}{e^2}\text{.}\) That means that \(\frac{1}{e^2}=1+\text{rate(decimal)}\text{.}\) Solving for the rate, we get that the rate (as a decimal) is \(\frac{1}{e^2}-1\text{.}\) To convert to a percentage, we just need to mulitply by 100. So, the rate is \(\left(\frac{1}{e^2}-1\right)100\)%. Since \(e\) is approximately 3,\(\frac{1}{e^2}\) is approximately \(\frac{1}{9}\text{,}\) so \(\frac{1}{e^2}-1\lt0\text{,}\) so this is a decay rate.

Example 14.22.

Let's find the end behavior of the exponential function \(f(x)=0.7e^{-x}\text{.}\) Since \(e^{-1}\lt 1\text{,}\) this function represents exponential decay, so the graph looks like the picture below:

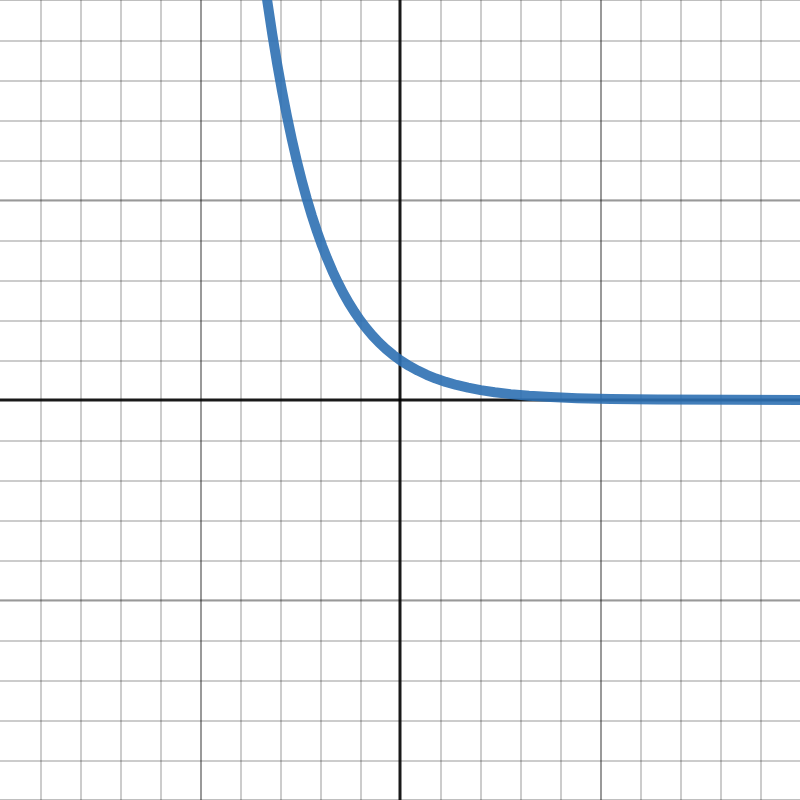
Therefore, we have the following end behavior:

  • As \(x\rightarrow \infty\text{,}\) \(y\rightarrow 0\)

  • As \(x\rightarrow -\infty\text{,}\) \(y\rightarrow \infty\)

Checkpoint 14.23.

Find the end behavior of each exponential function below:

  1. \(\displaystyle f(x)=5e^x\)

  2. \(\displaystyle g(x)=5e^{x+4}-8\)

Answer.

    • As \(x\rightarrow \infty\text{,}\) \(y\rightarrow \infty\)

    • As \(x\rightarrow -\infty\text{,}\) \(y\rightarrow 0\)

    • As \(x\rightarrow \infty\text{,}\) \(y\rightarrow \infty\)

    • As \(x\rightarrow -\infty\text{,}\) \(y\rightarrow -8\)

Solution.

  1. For \(f(x)=5e^x\text{,}\) since \(e \gt 1\text{,}\) this function represents exponential growth, so the graph looks like the picture below:

    Therefore, we have the following end behavior:

    • As \(x\rightarrow \infty\text{,}\) \(y\rightarrow \infty\)

    • As \(x\rightarrow -\infty\text{,}\) \(y\rightarrow 0\)

  2. For \(g(x)=5e^{x+4}-8\text{,}\) we have exponential growth, like the problem above, except that this function is shifted down by 8. So, we have the following end behavior:

    • As \(x\rightarrow \infty\text{,}\) \(y\rightarrow \infty\)

    • As \(x\rightarrow -\infty\text{,}\) \(y\rightarrow -8\)